Introduction

For a liquid rocket engine, turbopumps supply liquid oxidizer and fuel to the combustion chamber at high pressure. Turbopumps rotate at high speeds to reduce the rocket engine weight and achieve high power densities. Such requirement leads to cavitation in turbopumps. Therefore, to inhibit cavitation in the impeller, an axial type inducer is installed upstream of the turbopump. However, the inducer itself can suffer from cavitation. When the inducer cavitation becomes severe, its head coefficient, ψ, is decreased. Critical cavitation number (defined to be the cavitation number at which the head coefficient value decreases to 97 % of the nominal value) is used to signify the head coefficient degradation. Inducer cavitation can also cause hydraulic instabilities- rotating cavitation, asymmetric attached cavitation, and cavitation surge 17. The onset cavitation number is used to quantify the beginning of cavitation instability.

In cryogenic fluids or hot water, the temperature and vapor pressure within the cavitation bubbles are decreased due to the latent heat absorption by the bubbles from the liquid during the vaporization process. This phenomenon is referred to as the “thermal effect.” As the liquid temperature increases, the thermal effect is enhanced, and cavitation bubble growth is suppressed 02. 14 measured cavitation performance for pumps and inducers in liquid hydrogen. The critical cavitation number decreased with increasing liquid temperature or decreasing rotational speed. 11 found that the critical cavitation number and cavitation region length were decreased as the liquid nitrogen temperature was increased. 16 found that the critical cavitation number was reduced at high water temperatures.

07 observed the variations in cavitation region length at the inducer leading edge and the onset of cavitation instabilities with changes in the liquid (refrigerant R-114) temperature and rotational speed. As R-114 temperature increased, the leading edge cavitation region was shortened and rotating cavitation occurred at lower cavitation numbers. In R-114 (in which the thermal effect existed), the cavitation region was lengthened as the rotational speed increased, but the cavitation region length was insensitive to the rotational speed in cold water (in which the thermal effect was negligible).

18 investigated cavitation instabilities at different liquid nitrogen temperatures. They found the followings. First, rotating cavitation occurred at the same length of tip vortex leakage cavitation region regardless of temperature. Second, the onset cavitation numbers of rotating cavitation and asymmetric attached cavitation were decreased as the temperature of liquid nitrogen increased. Finally shaft vibration and rotating cavitation amplitudes were reduced at high liquid temperatures.

Alternatively, to quantify such thermal effects, two parameters have been suggested. 02 suggested a dimensional thermal parameter, Σ (m/s^3/2), from the Rayleigh-Plesset equation. Σ represents the amount of vapor pressure depression due to the thermal effect in a fluid ().

For cavitation in fluid machines, 08 derived a non-dimensional thermal parameter, Σ^* by nondimensionalizing the Rayleigh-Plesset equation (). For simplicity, they assumed inviscid flow.

In , Σ*=ΣC/U3 in which the bubble radius (R) and the distance (x) have been non-dimensionalized by the characteristic length C. C_p is the pressure coefficient, and σ is the cavitation number. In addition to the fluid properties of Σ, Σ^* incorporates the characteristic length (C) and reference velocity (U) of the pump representing the amount of the thermal effect on cavitation in fluid machines. In , as the temperature of the working fluid increases, the vapor density increases faster than the increase in the temperature. Thus, Σ is increased. According to , Σ^* is increased as the liquid temperature increases or the reference velocity decreases. In actual rocket engines using liquid oxygen, Σ^* ranges from 1.5 to 30 depending on the size and rotational speed of the inducer.

21 independently derived the non-dimensional thermal parameter Σ^* in their analysis of an inducer cascade and found that increasing Σ^* suppressed the onset of cavitation instabilities. 10 investigated the rotational speed effects using Σ^* in liquid nitrogen. The cavitation region length and critical cavitation number both decreased as Σ^* increased.

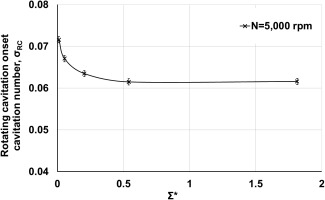
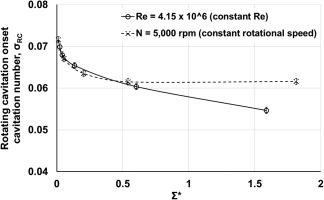
Hot water can be used as a surrogate test fluid for cryogenic oxidizer or propellant when it satisfies the similarity requirement in terms of the non-dimensional thermal parameter. 05 derived their DB parameter, which is a reciprocal of Σ^*, and they matched their DB value in water with that of liquid oxygen. In water, they found that decreasing DB completely suppressed rotating cavitation. 12 investigated rotating cavitation onset trends for varying Σ^* by changing the water temperature at a given rotational speed. The onset cavitation number decreased as Σ^* was increased for Σ^* < 0.54. However, for Σ^* ≥ 0.54, the onset cavitation number remained constant as Σ^* was increased (Figure 1).

Figure 1.

The rotating cavitation onset cavitation number versus the non-dimensional thermal parameter at 5,000 rpm and φ/φ_d = 1.0 12.

Previous researchers investigated the thermal effect on cavitating inducers by varying either the liquid temperature or rotational speed, but they did not consider viscous effects. However, when either the liquid temperature or rotational speed is changed, not only Σ^* but also the Reynolds number (Re = 2ρ₁rΩ²/μ₁) is simultaneously changed. In 12, as Σ^* was increased from 0.0116 to 1.80, the Reynolds number ranged from 2.6 × 10⁶ to 6.4 × 10⁶ as well. It indicates that Reynolds number possibly affects the occurrence of rotating cavitation. Yet, the individual effects of Σ^* and Re on the cavitation instability onset have not been examined. Therefore, the research objective of this study is to measure and understand the individual effects of Σ^* and Re on the onset of cavitation instability in a turbopump inducer.

Non-dimensional parameters

To identify the non-dimensional parameters governing cavitation growth in an inducer, the Rayleigh-Plesset equation for bubble dynamics is presented in 13.

Following 08, time have been normalized by x/U where x is the distance along the streamline followed by the bubble; U is the inducer blade tip rotational speed; and C is the inducer radius. Surface tension (S) and non-condensable gas effects (p_G0) are negligible after the initial stage of bubble formation 03. The resulting non-dimensional Rayleigh-Plesset equation is then:

In , Σ^* and Re are the two non-dimensional parameters which affect cavitation bubble growth. Increasing liquid temperature increases both Σ^* and Re. Increasing inducer blade tip velocity increases Re but decreases Σ^*.

Experimental apparatus and instrumentation

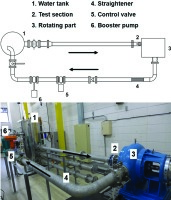
Figure 2 shows a schematic of the turbopump inducer water test facility at Seoul National University 12. The test facility has been designed to measure cavitating and non-cavitating inducer/pump performance over a wide range of operating conditions. The closed loop test facility includes a water tank, test section, flow straightener, control valve, and booster pump. A vacuum pump and compressed air supply pipe are connected to the water tank to control pressure. To adjust the water temperature, a 20-kW electrical heater has been employed in the water tank. The filtered water from the tank is circulated in the direction shown in Figure 2. Downstream of the collector, a flow straightener is located upstream of the flow meter for accurate flow rate measurement, and the control valve sets the flow rate. Farther downstream, the booster pump maintains a constant flow rate under cavitating conditions. Elastic couplings have been installed at the exit of the water tank and collector for alignment and vibration control. Rotating parts are composed of the test inducer, shaft, and motor. A 60-kW motor drives the inducer at rotational speeds of up to 10,000 rpm. The rotational speed of the motor is controlled to within ±1 rpm by a variable frequency drive.

Figure 2.

SNU turbopump inducer experimental facility.

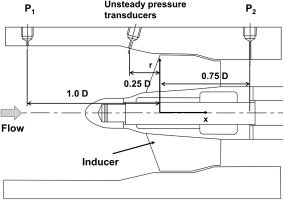
Figure 3 shows the test section instrumentation locations 12. Eight and four static pressure taps at x/D = −1.0 (P₁) and 0.75 (P₂) have been used to measure the inducer static head coefficient and cavitation number. Eight unsteady pressure transducers with an accuracy of 0.1 % of the full scale value (350 kPa) and 400 kHz frequency response have been installed at x/D = −0.25 to detect cavitation instabilities. Unsteady pressure signals have been sampled at 50 kHz for 1 second. Bulk water temperature has been maintained to within ±0.1 K during the test. The mean flow rate has been measured downstream of the flow straightener using an electro-magnetic flow meter with an accuracy of 0.25% of the full scale value (20 kg/s). The uncertainties of the head coefficient (ψ), flow coefficient (Φ) and cavitation number (σ) with a 95% confidence interval are ±0.00398, ±0.000422, and ±0.000759, respectively. All of the data presented in the paper have been acquired at the design flow rate condition.

Figure 3.

Test section schematic.

The test inducer (Figure 4) has been designed by the Korea Aerospace Research Institute (KARI), and its specifications are summarized in Table 1. The inducer has a high solidity and moderate blade tip angle at the inlet 04.

Figure 4.

Test inducer.

Experimental results and discussion

Cavitation instabilities

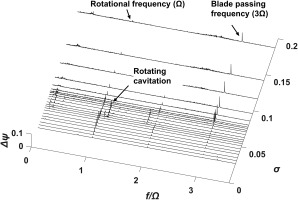
Fast Fourier Transform analysis and the cross-correlation of signals from two unsteady pressure transducers have been used to determine cavitation instability characteristics. Figure 5 shows the power spectral density plots of the unsteady pressure transducer signals for varying cavitation number 12. Three dominant peaks at f/Ω = 3.0 (σ > 0.072), 1.14 (0.053 < σ < 0.072), and 1.0 (σ < 0.053) are visible. According to 12, the peaks at f/Ω = 3.0, 1.14, and 1.0 correspond to blade passing frequency, rotating cavitation, and asymmetric attached cavitation, respectively.

Figure 5.

The power spectral density of inducer inlet pressure fluctuations (φ/φ_d = 1.0, Σ^* = 0.0116, Re = 2.58 × 106 [T_∞ = 298 K and 5,000 rpm]).

Rotating cavitation results in uneven cavitation regions rotating at a speed faster (super-synchronous) than the inducer rotational speed in the same direction. Thus, the frequency of rotating cavitation is f/Ω = 1.14 in the tested inducer 12. In the present study, the onset cavitation number of rotating cavitation (σ_RC) is defined to be the cavitation number at which the amplitude of the rotating cavitation frequency increases to five times its steady state amplitude. Repeatability for the onset of rotating cavitation has been confirmed by three separate tests, and σ_RC is repeatable to within 0.7% at the design flow coefficient.

Non-dimensional thermal parameter effects for constant Reynolds number

To investigate the effects of Σ^* for constant Reynolds number, Σ^* has been varied from 0.0227 to 1.55 while Re has been held constant at 4.15 × 10⁶. The test conditions are summarized in Table 2. From heat transfer analysis (Vergman and Incropera 2011), the temperature difference between the bulk water and wall varies from 0.04 K (308 K and 6,400 rpm) to 0.09 K (347 K and 3,500 rpm). The analysis procedure is described in the Appendix. The value of Σ^* = 1.59 is similar to Σ^* = 1.81 of the KARI liquid oxygen (LOX) inducer under the actual operating conditions (87 K and 20,000 rpm).

Table 2.

Test conditions for thermal parameter effects.

	Temperature [K]	Rotational speed [rpm]	Σ [m/s3/2]	Reynolds number	Σ*=ΣC/U3
Water	308	6,400	1.87 × 101	4.14 × 106	2.29 × 10–2
	313	6,000	3.14 × 101	4.18 × 106	4.21 × 10–1
	323	5,000	7.60 × 101	4.18 × 106	1.35 × 10–1
	337	4,000	2.43 × 102	4.13 × 106	6.03 × 10–1
	347	3,500	5.24 × 102	4.13 × 106	1.59
LOX	86.6	20,000	8.21 × 103	5.04 × 107	1.82

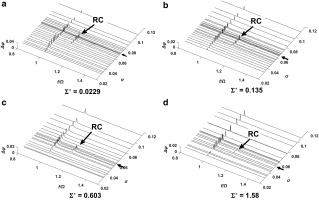
Figure 6 presents the power spectral density plots of the inlet unsteady pressure fluctuations for Σ^* ranging from 0.0229 to 1.59 at Re = 4.15 × 10⁶. The arrows indicate the onset of rotating cavitation, σ_RC. The onset cavitation number and magnitude of the rotating cavitation (RC) at f/Ω = 1.14 are decreased as Σ^* increases. Figure 7 shows the σ_RC plotted versus Σ^*. σ_RC monotonically decreases by 21% as Σ^* is increased from 0.0229 to 1.59. High-speed camera flow visualization qualitatively confirms this trend. Figure 8 shows the cavitation region on an inducer blade for Σ^* = 0.0229, and Figure 8 shows the same blade for Σ^* = 1.59 at σ = 0.081 and Re = 4.15 × 10⁶. The cavitation region for Σ^* = 1.59 is visibly smaller than that for Σ^* = 0.0229. According to 09, the onset of rotating cavitation depends on the length of the cavitation region. Thus, the visualization data are qualitatively consistent with the unsteady pressure data and confirm that increasing Σ^* suppresses the rotating cavitation onset.

Figure 8.

The inducer blade cavitation for Σ^* = 0.0227 (a) and 1.55 (b) (σ = 0.081, φ/φ_d = 1.0, Re = 4.15 × 106).

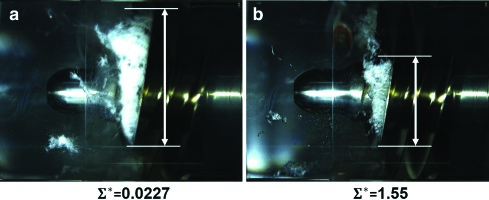
Figure 7.

The rotating cavitation onset cavitation number versus the non-dimensional thermal parameter comparing at Re = 4.15 × 106 and φ/φ_d = 1.0.

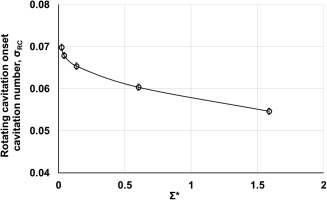
Figure 6.

The power spectral density of unsteady pressure fluctuation at various non-dimensional thermal parameter at Re = 4.15 × 106 and φ/φ_d = 1.0 for Σ^* = 0.0229 (a), 0.135 (b), 0.603 (c), 1.58 (d).

14 defined the minimum cavitation number, σ_min, which determines the cavitation bubble growth by considering the thermal effect () as

(5)

σmin=p∞−pv,min12ρlU2=σ+pv,∞−pv,min12ρlU2=σ+Δpv12ρlU2

where p_v,min is the minimum vapor pressure within the cavitation bubble. The minimum cavitation number is the s\

um of the cavitation number (σ) and the vapor pressure depression (Δp_v) normalized by the dynamic head (12ρlU2). As Σ^* is increased, Δp_v of the cavitation bubbles is increased, and σ_min increases. Thus, increasing Σ^* inhibits rotating cavitation onset.

Reynolds number effects for constant non-dimensional thermal parameter

Table 3 lists the test conditions for Re effects. Figure 9 shows the power spectral density plots at Re = 2.30 × 10⁶ (Figure 9) and 3.17 × 10⁶ (Figure 9) for Σ^* = 0.0125. Figure 10 shows the corresponding data at Re = 4.81 × 10⁶ (Figure 10) and 6.84 × 10⁶ (Figure 10) for Σ^* = 1.26. The arrows indicate σ_RC. For Σ^* = 0.0125, σ_RC remains constant as Re increases. For Σ^* = 1.26, σ_RC at Re = 6.84 × 10⁶ is higher than that at Re = 4.81 × 10⁶.

Figure 10.

The power spectral density plots of unsteady pressure fluctuations at Σ^* = 1.26 and φ/φ_d = 1.0 for Re = 4.81 × 106 (a) and Re = 6.84 × 106 (b).

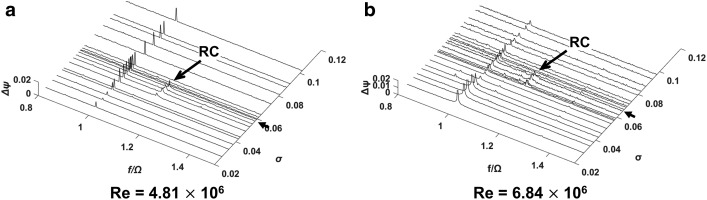
Figure 9.

The power spectral density plots of unsteady pressure fluctuations at Σ^* = 0.0125 and φ/φ_d = 1.0 for Re = 2.30 × 106 (a) and Re = 3.17 × 106 (b).

Table 3.

Test conditions for Reynolds number effects.

Temperature [K]	Rotational speed [rpm]	Reynolds number	Σ*
297	4,500	2.30 × 106	1.29 × 10–2
298	4,900	2.50 × 106	1.22 × 10–2
300.5	5,800	3.17 × 106	1.23 × 10–2
310	4,150	2.76 × 106	5.33 × 10–2
315	5,000	3.52 × 106	5.38 × 10–2
318	5,700	4.16 × 106	5.42 × 10–2
318	4,400	3.38 × 106	1.04 × 10–1
321	5,200	4.21 × 106	1.06 × 10–1
323	5,800	4.85 × 106	1.08 × 10–1
328	4,350	3.95 × 106	2.57 × 10–1
330.5	4,950	4.65 × 106	2.57 × 10–1
333	4,700	5.56 × 106	2.57 × 10–1
335	4,000	4.02 × 106	5.15 × 10–1
337.5	5,550	4.74 × 106	5.18 × 10–1
340	5,200	5.60 × 106	5.16 × 10–1
341	5,450	5.94 × 106	5.16 × 10–1
342	5,700	6.30 × 106	5.20 × 10–1
346.5	4,100	4.81 × 106	1.22
348	4,300	5.14 × 106	1.26
350	4,700	5.75 × 106	1.26
351.5	5,100	6.36 × 106	1.26
353	5,400	6.84 × 106	1.27

The dependence of σ_RC on Re for various values of Σ^* is presented in Figure 11. For Σ^* = 0.0125, σ_RC is independent of Re. On the other hand, for Σ^* ≥ 0.0537, σ_RC increases with increasing Re, and such Re effects (e.g., ∂(σRC)∂(Re)) become stronger as Σ^* increases. Visualization of the cavitation on inducer blades qualitatively confirms such Re effects. Figure 12 shows the inducer blade cavitation at Re = 2.30 × 10⁶ (Figure 12) and 3.17 × 10⁶ (Figure 12) for Σ^* = 0.0125. For Σ^* = 0.0125, the length of the inducer blade cavitation region is not affected by the Re change. For sufficiently low Σ^* (e.g., 0.0125), where the thermal effect is negligible [17], the vapor pressure depression is also negligible ((Δp_v ≈ 0). Therefore, σ_min () and σ_RC are insensitive to Re for low Σ^*.

Figure 12.

The inducer blade cavitation for Re = 2.30 × 106 (a) and 3.17 × 106 (b) (σ = 0.081, φ/φ_d = 1.0, Σ^* = 0.0125).

Figure 11.

The rotating cavitation onset cavitation number and the critical cavitation number for varying Reynolds numbers and Σ^* at φ/φ_d = 1.0.

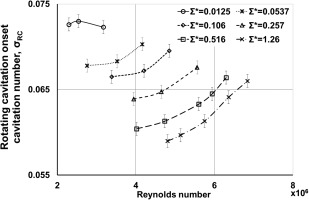
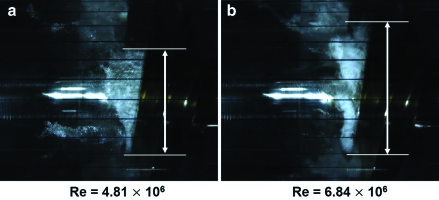
Figure 13 shows the cavitation region on an inducer blade for Σ^* = 1.23 at Re = 4.81 × 10⁶ (Figure 13) and 6.84 × 10⁶ (Figure 13). For Σ^* = 1.23, the length of the inducer blade cavitation region at Re = 6.84 × 10⁶ is visibly longer than that at Re = 4.81 × 10⁶. Thus, the rotating cavitation onset depends on Re at Σ^* = 1.23. 15 performed a numerical analysis of the Re effects on cavitation growth for a hydrofoil. They found that the cavitation region is bigger at a higher Re in liquid oxygen in which the thermal effect is significant. For high Σ^* values, where the thermal effect is significant, as Re increases, the dynamic head (12ρlU2) in increases, reducing σ_min. Thus, increasing Re promotes cavitation region growth, and the rotating cavitation onset occurs at a higher cavitation number. In typical rocket engines, Σ^* (1.5 ~ 30) and Re (1.0 × 10⁷~10⁸) are larger than the tested conditions. Thus, the effects of Σ^* and Re are expected to be more significant in real operating conditions.

Figure 13.

The inducer blade cavitation for Re = 4.81 × 106 (a) and 6.84 × 106 (b) (σ = 0.089, φ/φ_d = 1.0, Σ^* = 1.23).

Based on the current results, Figure 1 from 12 can be explained as follows. Figure 14 shows the combined plots of Figure 1 and Figure 7. In 12, experiments had been performed by varying Σ^* while maintaining a constant rotational speed of 5,000 rpm. Thus, Re increased from 2.6 × 10⁶ to 6.4 × 10⁶ simultaneously as Σ^* was increased from 0.0116 to 1.80. In Figure 14, σ_RC becomes independent of Σ^* for Σ^* > 0.54 at N = 5,000 rpm. On the other hand, σ_RC continues to decrease for Σ^* > 0.54 at Re = 4.15 × 10⁶. In the N = 5,000 rpm study 12, the cavitation suppression effects due to the increase in Σ^* and the cavitation promotion effects due to the increase in Re occurred simultaneously. For Σ^* < 0.54, the Re effects were weaker, and, thus, σ_RC was decreased as Σ^* increased. However, as Σ^* increased further, the Re effects became stronger, essentially cancelling out the Σ^* effects. Thus, σ_RC became “seemingly” independent of Σ^* for 0.54 < Σ^* < 1.80 for N = 5,000 rpm. However, when Re is kept constant at 4.15 × 10⁶ in the current study, σ_RC is decreased as Σ^* increases.

Figure 14.

The rotating cavitation onset cavitation number versus the non-dimensional thermal parameter for constant Reynolds number and rotational speed 12 at φ/φ_d = 1.0.

Conclusions

The individual effects of non-dimensional thermal parameter (Σ^*) and Reynolds number (Re) on rotating cavitation onset for a turbopump inducer have been measured in water. The new conclusions from this study are:

Nomenclature

Subscripts