Research facility and operating conditions

The data presented in this paper is based on experiments conducted in the axial turbine research facility LISA of the Laboratory for Energy Conversion (LEC) at ETH Zurich, as depicted in Figure 1. The test rig is a low-temperature, moderate-speed, continuously running rotating facility with non-dimensional parameter matching of real engine conditions. A radial compressor drives the air in the quasi-closed loop trough a two-stage water to air heat exchanger and a flow conditioning stretch before the test section. The turbine inlet total temperature is controlled within an accuracy of ±0.4 K. After the turbine section, the loop is open to the atmosphere, and the flow is then fed back through a calibrated Venturi nozzle for mass flow measurements to the compressor. The turbine shaft is connected to a reduction gearbox and a DC generator, breaking the turbine and controlling the rotational speed within an accuracy of ±0.02% (±0.5 rpm). The generated power is then fed back to the power grid. Because the air loop is open to the atmosphere, two rows of subsequent de-swirling vanes are installed to recover the static pressure back to ambient levels. A more detailed description of the rig and test section is given by (Behr et al., 2006).

Figure 1.

Overview of the turbine test facility “LISA” at ETH Zurich.

The investigated turbine configuration is a 1.5-stage, high-pressure turbine representative design. All blade rows and endwalls are 3D optimised geometries for reduced aerodynamic losses. The blade count for stator 1, the rotor, and stator 2 are 36, 42, and 36, respectively.

Compressor air from the main loop is bypassed and used as cavity purge flow in the rotor upstream cavity. The air is extracted before the flow stretch, and injected through 10 tubes in the first stator vanes into the cavity below. The purge flow injection rate (IR) from the cavity into the main annulus is defined as follows:

The purge air is calculated from the mass flow balance of extracted bypass flow and cavity sealing leakage flow through the drum, normalised by the total turbine mass flow (Figure 2). Both mass flow rates are measured with calibrated standard nozzles.

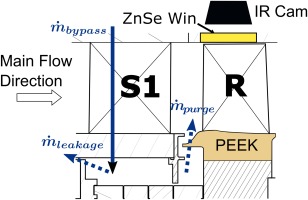
Figure 2.

Schematic cut of first stage with purge flow injection path and optical access to the rotor endwall.

For this study, the turbine was operated at the constant design pressure ratio of Π1.5=1.65, accounting for changes in atmospheric pressure. Further relevant operating parameters are summarised in Table 1.

Rotor endwall and cavity sealing geometry

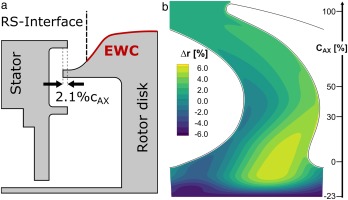
The rotor upstream cavity sealing is an overlapping angle-wing type with 2.1% cax of overlapping (Figure 3b). Additionally, a radial sealing arm separates the injection plenum from the rotor disk. This sealing geometry is representative of real engine designs.

Figure 3.

Rotor stator hub cavity sealing configuration (a) and hub endwall contouring (b).

The non-axisymmetric rotor hub endwall contouring is extended upstream into the rotor sealing arm arrangement. The contouring starts at −23% cax upstream of the rotor leading edge, at a radial location inside the sealing arrangement below the nominal hub radius of 330 mm. The contouring consists of a pronounced hill on the pressure side of the passage next to a trough at the suction side, near the blade leading edge axial location (Figure 3b). As shown, most of the contouring is located upstream of the leading edge until reaching 50% of the axial chord. The rotor blades have a constant fillet radius of 3 mm.

Design intention of rotor endwall

The design of the rotor endwall contouring is based on a CFD optimisation cycle, which only uses the 1.5-stage aerodynamic efficiency as an optimisation target, with mass flow as a constrain. The design steps were as follows:

This toolchain was run with AutoOpti by DLR, using a generic algorithm for the generation of designs and a surrogate model based on kriging. In total, 89 design parameters were used for the aerofoil and endwall. The optimisation required approximately 1,500 members to converge. More details about the optimisation algorithm are given in (Aulich and Siller, 2011).

A difference in efficiency of 0.3 percentage points in 1.5-stage efficiency compared to flat endwall was achieved in the optimisation. However, as the aerofoil and endwall were optimised in combination, it was difficult to isolate the effect of the EWC itself. Compared to a flat endwall, a more uniform mass distribution of the purge over the circumference was observed. Further, the cross-passage migration of the pressure side horseshoe leg shifted downstream, and the passage vortex and suction side horseshoe vortex leg remain separated. This resulted in two small pressure loss cores, compared to one big loss core without contouring.

Experimental setup

Heat transfer measurements in the facility were performed with an infrared based technique described by (Lazzi Gazzini et al., 2017b) and (Laveau et al., 2014). A Flir IR SC7300L camera was used to record the temperature of the rotor endwall through infrared transparent Zinc-Selenide (ZnSe) windows installed in the rotor casing (Figure 2). The camera was mounted on a traversing system, allowing vertical adjustments and different camera angles using various angle shims to extend the optical coverage through the window.

A quasi iso-energetic setup was used to create controlled boundary conditions at the region of interest. Therefore, a custom-made thin film surface heating insert was manufactured and installed on the rotor. The insert was manufactured from PEEK (Polyether-ether-ketone), a thermally low conductive, high strength, base substrate, replicating the exact endwall geometry. Pt100 temperature sensors were installed in the insert 1.5 mm below the surface to account for conduction losses. The surface was coated with an 1.4 µm thick layer of Nickel using a direct chemical deposition technique on the PEEK, and pulsed laser ablation was used to create a serpentine like heating track with a uniform width of 1.9 mm. The heating track on the endwall is depicted in Figure 4a, also indicating the achieved heated area in red. The inherent need for track turns resulted in unheated areas close to the blade fillet, which were therefore excluded in the post-processing. For improved infrared signature and reduction of reflections, all rotor surfaces were painted with Nextel black velvet high emissivity paint (ε=0.98 (Kwor and Matteï, 2001)).

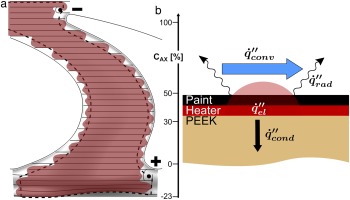
Figure 4.

Endwall heating track design with indicated heated area (a) and energy balance of the setup (b).

Nonuniformities in heat flux creation were corrected during post-processing with the non-uniformity factor Sq derived from transient heating tests, as described by (Lazzi Gazzini et al., 2017a) and defined in Equation 2. This local factor accounts for differences in electrical resistance or current concentrations on the heater.

The temperature sensor data acquisition and control of the heaters was accomplished with a rotating data acquisition system mounted on the rotor disk, controlled over a wireless connection (Mansour et al., 2015). The electrical power for the heating platforms (and for the rotor on-board data acquisition system) was transferred to the rotating system via a two-channel slip-ring. The heater supply current was measured and recorded externally.

Image processing

Measurements were performed for a specific stator 1 to rotor relative position. A shaft mounted optical triggering system was used to trigger the infrared camera acquisitions, and images were recorded with a sensor integration time of 10 µs to reduce the blurring due to platform movement. For extended optical coverage of the endwall, three different camera tilting angles were used (−4.5°, +4.5°, and +15°), and a sequence of 1,000 individually triggered images were recorded for each position. All frames of an image sequence were registered with respect to a reference, and only images within a ±1 pixel shift were considered for post-processing. Three different platform power levels, with an averaged produced heat flux between 3.3 and 9.2 kW/m², were used for the calculation of heat transfer quantities.

Silver infrared reference markers were used on the endwall to project the two dimensional image data on the three dimensional endwall geometry by geometrical transformation.

Heat transfer calculation

The convective heat flux is calculated from the overall energy balance (Equation 3) on the heater surface, schematically depicted in Figure 4b.

The electric heat flux is produced by Joule heating (Equation 4) and directly calculated from the supply voltage and current and corrected for local non-uniformity by the factor Sq. The radiative heat flux is calculated according to Equation 5 with the emissivity ε of the paint and σ being the Stefan-Boltzmann constant. The conduction losses are calculated using the installed temperature sensors inside the heater, according to the one dimensional conduction Equation (6) and based on the thermal properties of the substrate.

The conduction losses were calculated for every heat flux step, and averaged over the passage for the energy balance.

For each heating step, the convective heat flux and wall temperature were obtained. A least square linear fitting was used to calculate the local heat transfer coefficient h and adiabatic wall temperature Taw (as defined in Equation 7) from the three heating steps.

Numerical setup

A multi-block structured mesh was generated for the 1.5 stage configuration (including cavities). The mesh consisted of approximately 11.6 million nodes, which provided a high-quality boundary layer resolution. The distribution of the non-dimensional wall distance y+ for the simulation domain is shown in Figure 5.

Figure 5.

Non-dimensional wall distance for CFD simulations.

The numerical investigation was performed with the commercial solver ANSYS CFX version 17.0. Steady state computations were conducted using the high-resolution advection scheme and the total energy equation, including viscous work terms for compressible flow. Computations were performed with the SST turbulence model with the γ-θ transition model and mixing plane for the stator-rotor interface.

The surface roughness from the experiments was modelled using the roughness model supplied in CFX. Details about the model are described by (Lutum et al., 2015). Local roughness quantities were derived by profilometer measurements along the blade endwall. From this investigation, an equivalent sand grain roughness level of 20 µm was determined and applied for the current predictions. The spatial discretisation was quasi-second-order for all investigated cases.

Inlet profiles for total pressure, total temperature, and turbulence quantities were obtained from corresponding experiments. The purge flow boundary conditions were specified by a mass flow rate of IR = 0.8% and total temperature of 328 K. Numerical heat transfer results were determined from a set of computations consisting of an adiabatic and heat flux calculation for the same operating conditions.

Measurement uncertainty

The measurement uncertainty evaluation was performed by successively perturbing the input parameters in the post-processing routine as sequential perturbation, as described by (Moffat, 1988). The local distribution of the Nusselt number and non-dimensional adiabatic wall temperature uncertainty for the design case are presented in Figure 6.

Figure 6.

Uncertainty distributions of relative Nusselt number (a) and absolute adiabatic wall temperature (b).

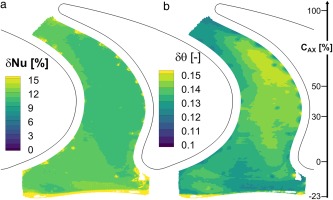
For the measurements presented, the platform averaged relative uncertainty was reported to be ±12.0% for the Nusselt number, and an absolute uncertainty of ±0.14 [-] for the non-dimensional adiabatic wall temperature. The main contributors to the Nusselt number uncertainty were the conductive losses (67.9%), the surface temperature (27.2%), and non-uniformity scaling factor Sq (3.5%). For the adiabatic wall temperature, the uncertainty in surface temperature (70.3%) and conduction losses (29.7%) were the main contributors. These uncertainty contributions are summarised in Table 2.

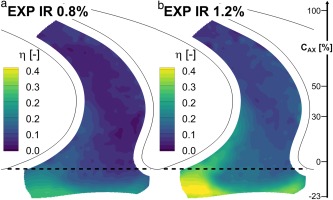
Endwall heat transfer for design point operation

Figure 7 shows the Nusselt number and adiabatic wall temperature distribution on the hub endwall for the design purge condition IR = 0.8%.

Figure 7.

Nusselt number (a) and adiabatic wall temperature (b) distributions for design purge flow rate (IR 0.8%).

The small band of very high heat transfer at the beginning of the heated platform was consistent with results reported previously with a similar measurement setup (Lazzi Gazzini et al., 2017b; Laveau et al., 2013), and arose from the start of the thermal boundary layer. A wedge like region of increased heat transfer followed at the mid-passage position, extending to approximately 10% cax from the leading edge measured at the mid-span (region A). This region coincides with the hill of the endwall contouring (Figure 3b) and can be explained by the induced acceleration of the flow. In contrast, lower heat transfer was observed towards the leading edge suction side of the blade, following the endwall trough. The isolated small region upstream of the leading edge, with very low heat transfer, was introduced by the electrical connection of the thin film heater, which was neglected in the analysis. Moreover, an increase in heat transfer was observed towards the throat region, which was attributed to the flow acceleration.

The adiabatic wall temperature (Figure 7b) decreased through the rotor passage. This was expected, as the flow temperature decreases due to expansion and acceleration. As the bypass air was extracted upstream of the turbine, the injected purge flow had a higher temperature than the main flow. Therefore, contrary to cooling in real gas turbines, the purge flow was heating the endwall in this measurement setup. The very start of the platform exhibited the highest adiabatic wall temperature over the full pitch, with iso-lines closely following the shape of the endwall contouring (Figure 3b).

As this part of the endwall extends inside the cavity and below the hub radius of the upstream NGV, it can be concluded that the changes seen in this region are associated with purge flow interaction. The extent of increased adiabatic wall temperature at the suction side (up to the 30% axial chord) indicates that a high amount of hot purge air is entrained into the suction side horseshoe vortex, whereas the rapid decrease over the endwall hill indicates the presence of the main flow.

Comparison with CFD predictions

The predictions from CFD simulations were compared to the experimental data by means of the endwall resolved Nusselt number and non-dimensional adiabatic wall temperature distributions, and the lateral averaged data of these values.

The simulation results for design purge flow rate are depicted in Figure 8. The increase of local heat transfer at the endwall hill upstream of the leading edge was clearly captured by CFD, which was visible as a wedge-like region (region A). The effect of the horseshoe vortex at the pressure side leading edge (region B) was not as clearly visible in the experiments as in CFD. However, a local increase of heat transfer was observed in both cases. No influence of the horseshoe vortex on the suction side was visible for both the experiments and the simulation, as the effect was too close to the blade and therefore not covered by the heated surface of the measurement setup. The adiabatic wall temperature exhibited two locations of purge entrainment into the passage with increased temperature (regions A and B). The larger region following the side of the endwall, which extended up to 30% cax, was similar to the hot streak in the experiments (Figure 7b) except for a slight pitch-wise shift. The second region (B) was not visible in the experiments. The relevant patterns for both quantities were captured in the simulation.

Figure 8.

Distributions from CFD simulations of Nusselt number (a) and adiabatic wall temperature (b) for IR 0.8%.

The lateral averaged results are compared in Figure 9. A general agreement within ±15% for the Nusselt number was found, although the high heat transfer region at the start of the heater was not captured by the simulations. Further, the increase through the passage was predicted to be less pronounced and start farther downstream than measured in the experiment. The trends for the adiabatic wall temperature were captured with a change in slope at approximately −5% cax. The offset in adiabatic wall temperature in the simulation, especially at the beginning of the platform, is explained by a miss-match in purge flow temperature and a different heat pickup compared to the experiments.

Figure 9.

Lateral averaged Nusselt number (top) and adiabatic wall temperature (bottom) for CFD and experiments (IR 0.8%).

Purge flow influence on endwall heat transfer

The effect of purge flow on the endwall heat transfer was investigated with three different injection rates. Accordingly, the design point IR = 0.8% case was the reference, and compared against zero injection IR = 0.0% and a high injection rate IR = 1.2%. This was accomplished by means of the relative Nusselt number difference, defined as follows:

Figure 10 shows the relative difference to nominal injection for both off-design cases. A general observation reveals that for both cases, there were local changes in heat transfer up to ±20%. However, significant changes were only found within the first 30% of the axial chord length. This implies that after the passage cross flow hits the blade suction side, all the purge flow is mixed into the secondary flows and detached from the endwall.

Figure 10.

Relative difference in local Nusselt number for IR 0.0% (a) and IR 1.2% (b) compared to design point (IR 0.8%).

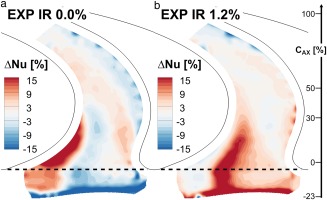
In the case of zero purge injection, a distinct increase of heat transfer was observed along the suction side, from the leading edge downstream to approximately cax=0.3. This implies that the purge flow in the reference case interacts with the suction side horseshoe vortex, which usually enhances the local heat transfer due to increased shear. However, for the investigated geometry with endwall contouring extending into the cavity, the purge flow exited the cavity close to the blade suction side, as shown in Figure 11a by CFD. The low momentum purge flow was reducing the local flow velocity, and therefore the heat transfer. Over the endwall hill region, a slight decrease in heat transfer was observed for no purge flow. Additionally, the trace of increased heat transfer (starting from the pressure side leading edge over the endwall to approximately 50% cax) indicates a stronger influence of the horseshoe vortex migration for the case without purge flow injection.

Figure 11.

Purge flow stream lines (top) and relative yaw angle contour (bottom) for IR 0.8% (a) and IR 1.2% (b).

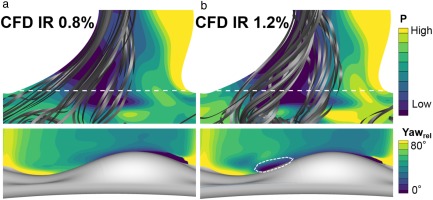
For the case of high purge flow rate, increased heat transfer was observed in a triangular region extended up to 35% of the axial chord (where it ends at the blade suction side). The highest increase (up to 20%) was located in a band like region starting from the beginning of the platform along the side of the endwall hill.

For IR = 1.2%, the purge flow was entrained as two different jets into the main flow and endwall (Figure 11b). Due to the higher purge momentum, the relative yaw angle of the main flow was reduced by the purge, and enhanced mixing of both flows was present. Additionally, the purge entrainment at the suction side caused a mass redistribution and flow acceleration of the main flow in the region between the two purge jets.

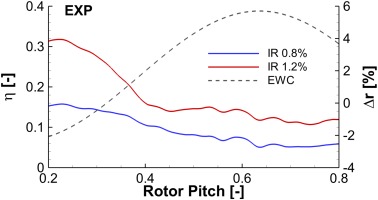
The influence of purge rate on the pitch wise distribution of absolute heat transfer over the passage is shown in Figure 12, for the indicated dashed line in Figure 10. Towards the suction side, both purge flow rates of 0.8% and 1.2% exhibited near identical levels of heat transfer, whereas the zero purge rate exhibited the same trend but with elevated levels. This reduction in heat transfer was caused by the local reduction in velocity due to the presence of low momentum purge air, as described previously. Between the 0.3 and 0.6 rotor pitches, the heat transfer was the highest modulated due to purge flow. Moreover, the most uniform circumferential heat transfer distribution on the endwall was achieved without purge flow. The modulation due to purge flow was similar for both injection ratios (0.8% and 1.2%), with different amplitudes at the maximum level leading to an increased non-uniformity over the pitch.

Figure 12.

Pitch-wise Nusselt number distribution and endwall radius change at indicated location in Figure 10.

Purge flow endwall cooling effectiveness

The cavity purge flow injection affected the main and secondary flow structures in the rotor passage, and provided cooling of the endwall due to the temperature difference compared to the main flow. This cooling was evaluated by means of a cooling effectiveness η, as defined in Equation (11), where the adiabatic wall temperature of the zero purge flow case was taken as a reference.

The difference in adiabatic wall temperature was normalised by the difference between the purge flow temperature Tc (measured in the cavity upstream of the radial sealing arm) and the rotor inlet relative total temperature.

Figure 13 shows the cooling effectiveness for both nominal and high purge rates for the rotor endwall. The general pattern is the same for both cases. The highest cooling effect was observed at the very beginning of the platform, where the endwall is below the nominal main flow hub radius (and therefore immersed in the purge flow). The other area of purge cooling started at the EWC trough and extended up to 30% of the axial chord along the blade suction side. This pattern was very similar to the regions of decreased heat transfer due to purge flow injection discussed in the previous section (Figure 9). This further confirms that the purge flow is predominantly swept to the suction side of the blade. This concentration is also illustrated in Figure 14, which shows the circumferential distribution for the same axial position indicated in Figure 13. The effectiveness is approximately twice as high at the suction side with the endwall trough compared to the rest of the pitch.

Figure 14.

Pitch-wise cooling effectiveness distribution at indicated location in Figure 13.

Figure 13.

Experimental endwall cooling effectiveness for IR 0.8% (a) and IR 1.2% (b).

The cooling effectiveness was approximately 75% higher for the high injection case (over the design injection case), where the overall platform averaged effectiveness was 0.085 for IR = 0.8%, and 0.150 for IR = 1.2%.

Variables

Greek

Subscripts

Abbreviations